Nonlinear Control of Gynmastic Robots

1. Objective

2. Four-link planar gymnastic robot

1) Overview

This study aims to realization of gymnastic robots, which can perform jumping, somersault, and back handspring. Back handspring is one of the difficult motion task that has never performed by real machines (Fig. 1).

At the first step, we try to derive feedback controller. When human performs gymnastic motion, learning and its feed-forward control may plays dominant role. However, as long as the learning is based on sensory information, feedback controllers can give important insights into the learning mechanism, especially when the motion is generated only by feecback controller. Moreover, feedback controllers have their own advantages over feed-forward ones; a robustness against sudden change of environment is the one of them.

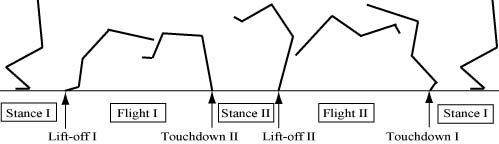Fig. 1 Back handspring motion

2) Model

Figure 2 shows a newly developed planar gymnastic robot. The robot has four links, serially connected by three joints. Each joint is actuated by geared servomotor (Maxon 20 W) through a timing belt. Most of the mechanical parts are machined out of aluminum frame. Overall height of the robot is 0.46 m and the total weight is about 1.74 kg.

Figure 3 shows the mathematical model. The dynamics are described by Lagrange's equation of motion and Lagrange's impulse equation with constraints.

Fig. 2 Mechanical model	Fig. 3 Mathematical model

3) Gymnastic controller

Back handspring is a rapid and complex motion task composed of multiple phases. In the control problem, it is not realistic to depend on some pre-planned reference trajectories, because in general such a trajectory tracking scheme cannot adapt to the changes of enviromnent, which are difficult to predict. Instead, we try to achieve complex motion tasks by describing simple target dynamics (equation of motion) about global physical quantities such as Center of gravity (CoG) or momentum.

The target dynamics we chose are:

• Vertical dynamics: simple oscillation of the vertical position of CoG
• Horizontal dynamics: constant acceleration of the horizontal position of CoG
• Rotational motion: regulated angular momentum around CoG along to the horizontal position

4) Simulation

• Simulation of vertical oscillation [AVI 738K]

• Simulation of successive back handspring [MPG 1,824K]

5) Experimemt

• Experiments on back-handspring and somersault [MPG 1781K]

• Back-handspring [MPG 547K]

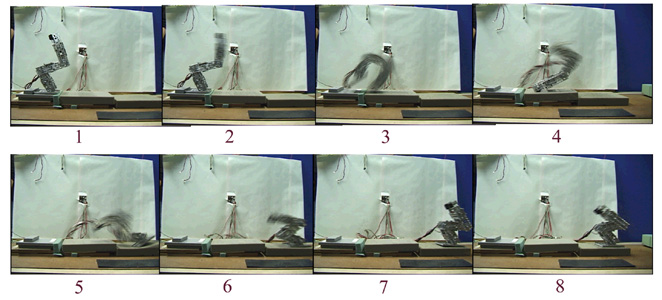Fig. 4 Snapshot of back handspring

3. Time-optimal control of two-link robot

1) Overview

In this study, we derive the analytical time optimal posture control law for free flying planar objects having non-zero angular momentum. The system is described by an affine nonlinear system with the drift term having no equilibrium.

The obtained time optimal solutions in this study include simple closed form formulae of the control law. They also show that the problem leads to a singular optimal control problem depending upon the initial posture; the switching time is once when the singular solution does not occur while is twice when the singular solution is used.

Fig. 5 Two-link free-flying robot	Fig. 6 High bar diver simulation

2) Simulation

As an application, the somersault motion of a diver approximated by the two link system is simulated. Since the optimal control solution is analytically known, online optimal path planning is very easy.

• Fly away from high bar [AVI 145K]

• Long jump of Acrobot [AVI 292K]

4. Reference

• Hyon, S., Yokoyama, N. and Emura, T., Back handspring of a multi-link gymnastic robot - reference model approach, Advanced Robotics, vol. 20, no. 1, pp.93-113, 2006.

• Hyon, S., Yokoyama, N. and Emura, T., Back handspring control of a multi-link gymnastic robot, submitted to 3rd IFAC Symposium on Mechatronic Systems , pp.73-78, 2004.

• Mita, T., Hyon, S. and Nam, T., Analytical time optimal control solution for a two link planar acrobot with initial angular momentum, IEEE Trans. on Robotics and Automation, pp. 361-366, 2001.